0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, 610, 987, 1597...
By definition, the first two numbers in the Fibonacci sequence are 0 and 1, and each subsequent number is the sum of the previous two.
F(n)=F(n-1)+F(n-2)
F(0)=0, F(1)=1
They are intimately connected with the golden ratio; for example, the closest rational approximations to the ratio are 2/1, 3/2, 5/3, 8/5, ...
Let us try to visualize glden ratio using our fractals.As the size of the parties will use the Fibonacci numbers.
If the majority party - even, and less - not even, we get this pattern:
If the majority party - not even, and less - even, we get this pattern:
If the two sides do not even - we get a symmetrical pattern:if:
Then:
if:
Then:
As you can see, fractal repeats part of the overall fractal increased by
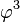
This method of constructing fractals can be considered as the visualization of irrational numbers.Irrational numbers are those real numbers that cannot be represented as terminating or repeating decimals. Fractal appears when the ratio of the rectangle -> irrational. (Or, if one side is 1 and the second = irrational number). If the size of the parties - are relatively prime - we have a rational approximations of real numbers.
By analogy with the iterative fractals. Only in the iterative fractals detailing increases, with more iterations, and in these fractals - if the ratio of the size of the parties seeks to irrational number (the length of the period decimal point goes to infinity).
In addition, exactly the same fractals are obtained, in the squares, if you use other angles.
